When I was an undergraduate, one of my professors told the following funny (and probably apocryphal) anecdote (recalled from memory):
A court case was being tried in New Mexico. A group of pornographers were charged with smuggling pornography from Mexico by projecting it across the border to a camera. The defense argued that nothing physical was transported, and in the end the argument boiled down to this: if light moved at a finite speed, the films were being transported; if it moved at infinite speed, the defense was correct. A physicist was brought in to discuss the speed of light but, after a number of figures were presented, the judge interrupted. “When I put my hands over my eyes, the light stops coming immediately, and when I move my hands, it reappears instantly. The speed of light is infinite – the defendants are not guilty!”
The reason I suspect this story is apocryphal is that science has accepted that the speed of light is finite – albeit very large – for centuries. The value, usually denoted c, is approximately meters/second, or 186,282 miles/second. In fact, as we will see in later posts, light is the fastest thing in the universe. The topics we address in this post: a brief history of measuring the speed of light, and how these measurements led inexorably to Einstein’s special theory of relativity.
We will, in fact, be concerned with two distinct classes of measurements. The first class are techniques for measuring the value of c itself. The second class are attempts to measure the change in the speed of light due to relative motion of the source or observer. We start with the first of these, and then discuss the reasons for the second class of measurements.
Many early ‘scientists’ or ‘natural philosophers’, though not all, imagined light to be of infinite speed. Aristotle was a proponent of this view, in large part because of his flawed idea that sight was a process of emission from the eye. Though some scientists challenged this idea (notably Ibn al-Haytham), the infinite speed seemed to be the prevailing view for most natural philosophers for quite some time.
One of the first attempts to experimentally measure the speed of light was undertaken by Galileo. The technique was simplicity itself: an experimenter would unveil a lantern on a mountaintop, an observer at a distant point would unveil his own lantern at the sight of the first lantern, and the first observer measures the amount of time before the second signal arrives. This is illustrated below:

This experiment produced a result indistinguishable from infinity, though it is not difficult in hindsight to understand why. The round-trip time for light traveling a distance d between mountaintops is
.
If we conservatively assume that Galileo had observation points a mile apart, and that the second observer instantaneously unveils his own lantern, the signal will return to Galileo in seconds! Considering human response time is on the order of 0.1 seconds, it’s clear that Galileo could not detect this time delay. One of the longest straight-line paths on the Earth is from Uncompaghre Peak, Colorado to Mount Ellen in Utah, a 183-mile separation. Galileo would fare no better with this distance;
seconds. Even if we get unrealistically optimistic and imagine using a system of mirrors to create a path around the circumference of the Earth (24,900 miles),
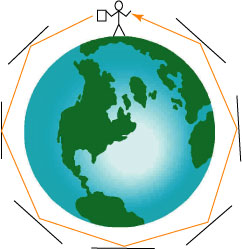
we still find a time delay seconds, still not within measuring capabilities of an unaided human.
In modern terms, I would say that the speed of light pwned Galileo! He realized, though, as others did, that his negative result simply meant that the speed of light could be much faster than he could detect.
The first successful measurement of the speed of light was apparently made by Ole Christensen Römer (1644-1710), a Danish astronomer. In the 1670s, Römer was studying eclipses of Jupiter’s moon Io, which should presumably appear at near regular intervals because Io is moving in a circular orbit around Jupiter. Römer observed, however, that the time between eclipses varied, and in fact came slightly less frequently when Earth is moving away from Jupiter, and slightly more frequently when Earth is moving towards Jupiter. He concluded (as did his supervisor Cassini before him), that this variation arose from the finite speed of light.
How does this work? Let us quote from the English translation of his 1677 paper (figure from Wikipedia, from Römer’s original paper: note the ‘smiley face’ in the Sun!):

Now, suppose the Earth, being in L towards the second quadrature of Jupiter, hath seen the first satellit at the time of its emersion or issuing out of the shadow in D; and that about 42 1/2 hours after (vid. after one revolution of this satellit,) the Earth being in K, do see it returned in D; it is manifest, that if the Light require time to traverse the interval LK, the Satellit will be seen returned later in D, than it would have been if the Earth had remained in L, so that the revolution of this Satellit being thus observed by the Emersions, will be retarded by so much time, as the Light shall have taken in passing from L to K…
Let us try and illustrate this a bit more simply below.

Suppose Jupiter starts out a distance from Earth at the beginning of one of Io’s eclipses (eclipse 1), that Io makes a full revolution of Jupiter in time T, and that Jupiter is moving away from Earth at velocity v. By the time the next eclipse begins (eclipse 2), Jupiter is now a distance d = vT further away from Earth than it was during eclipse 1. The light from eclipse 2 must travel a distance d = vT further than the light from eclipse 1 needed to travel, and will therefore arrive ‘late’ by a time
.
The apparent duration of the orbit of Io is increased by ! Similarly, when Jupiter and Earth are moving closer together, the apparent duration is decreased by
. If we know the period of Io (or its average value), and we know the relative speed difference between the Earth and Jupiter, we can experimentally calculate the value of c! The change in a single orbital period is small, but Römer looked at the cumulative change over a large number of orbits (40, according to his paper), and found a measurable deviation of 22 minutes. Römer himself did not explicitly calculate the speed of light in his work, but others (including Dutch smarty-pants Christiaan Huygens) did, and estimated the speed within 20% of the current accepted value.
The astute observer may notice a similarity between Römer’s calculation and the Doppler shift, in which the frequency of a wave signal is increased/decreased as the source moves towards/away from the observer, respectively. In fact, Römer’s technique is a simple application of a Doppler shift; the observed angular frequency of Io as measured on Earth is, according to the equation above,
,
which is exactly the classical Doppler formula for a slowly moving source.
We skip ahead now to the work of Hippolyte Fizeau (1819-1896), who in 1849 designed a new technique to measure the speed of light. In essence, it is a reimagining of Galileo’s unsuccessful experiment, and is illustrated schematically below:
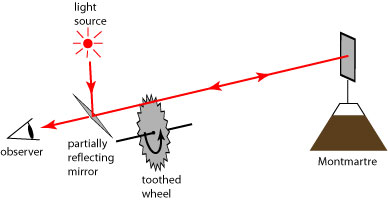
Light leaves a source, is reflected off of a partially reflecting mirror, and illuminates the spokes of a rapidly rotating toothed wheel. The teeth of the wheel break the light up into ‘pulses’, each of which travel to a distant mountaintop and are reflected by a mirror there. If the pulse returns while a gap in the teeth is present, the pulse passes through and reaches the eye of the observer. Knowing the distance to the mountain, the number of teeth on the wheel and the frequency of rotation at which the returning pulse reaches the eye, one can accurately measure the speed of light.
Fizeau’s results were published in Comp. Rend. Acad. Sci. (Paris) 29 (1849), 90-92. (This reference was extremely hard to pin down, and I’m going to reprint it and a translation in its entirety in another blog post.) How is this an improvement over Galileo’s mountain to mountain experiment? To quote from (a Babelfish-translation of) Fizeau’s own words,
When a disc turns in its plan around the centre of face with a great speed, one can consider the time employed by a point of the circumference to traverse a very small angular space, 1/1000 of the circumference, for example.
When the number of revolutions is rather large, this time is generally very short; for ten and hundred turns a second, it is only 1/10000 and 1/100000 of second. If the disc is divided has its circumference, with the manner of the toothed wheels, in equal intervals alternatively empty and full, one will have, for the duration of the passage of each interval by the same point of space, the same very-small fractions.
During such short times the light traverses limited enough spaces, 31 kilometers for the first fraction, 3 kilometers for the second.
The fast moving disc takes the place of the slow human reaction time. Supposing the wheel has N teeth, the gaps in the teeth are separated by an angle
.
(Using radians =360 degrees.) The time for a pulse to traverse the distance to the mountain and back again is
.
A bright spot will appear on the observation screen if the disc has rotated to the adjacent gap at exactly the time the pulse returns; the ‘magic’ angular frequency is given by
;
in terms of rotations/second, this becomes:
.
Solving this equation for c gives us
.
What estimate can we get with Fizeau’s results? Fizeau used a disc with 720 teeth. The experiment was done with two posts, one at “a house located at Suresnes, the second on the height of Montmartre, at an approximate distance of 8633 meters.” The first appearance of a luminous point occurred at turns/second. Plugging these numbers into our equation, we get a result of
m/s,
which is roughly 4% off the actual value!
Let us now turn our attention to the second class of measurements: measuring changes in the speed of light due to the Earth’s motion. As we discussed in our previous relativity posts (pre-history, Newtonian relativity), by the late 1800s scientists had realized that there was something problematic when combining Newtonian relativity (the laws of motion are the same in all inertial reference frames) with Maxwell’s equations (the laws which describe light and electromagnetic fields). In particular, one can easily use Maxwell’s equations and Newtonian relativity to show that observers in different reference frames will predict different forces between electric charges! As we’ve stated in previous posts, this would be comparable to saying that a stationary witness to a car accident sees both cars totaled while a moving witness claims it was only a fender-bender!
This was a clearly at odds with reality, and so physicists were forced to rethink their views. Clearly only one of the (potentially infinite) number of observers can be correct about the forces, but which one?
The (incorrect) answer came by analogy. At that point, all waves known to science required a medium of some sort to travel through: sound waves propagate through air, water waves travel through water, string vibrations travel along strings. It was perfectly reasonable at the time to assume that light, an electromagnetic wave, must also be traveling through some as yet unobserved medium. This medium was dubbed the aether or luminiferous aether. An observer at rest with respect to this aether would see all light waves traveling at c, but observers in motion with respect to the aether would measure different speeds for light, depending on their motion relative to the light. For instance, according to Newtonian relativity, an observer moving at velocity v parallel to a beam of light would measure the speed of the light beam as , while an observer moving at velocity v antiparallel to the beam would measure its speed as
.
The biggest problem with the idea of an aether is that nobody had ever observed it. It had to be robust enough to carry high-frequency light waves but tenuous enough that its presence was otherwise unobservable. The Earth, however, was already known to move at 30 kilometers/second in its path around the Sun. It would have to be moving, at some point in its orbit, at least at this speed relative to the hypothetical aether. Even though nobody knew how to make a direct measurement of aether, one could presumably indirectly detect it by measuring changes in the speed of light as the Earth moves.
Therein lies another problem, though: the speed of light is km/s, while the speed of the Earth is only 0.01% of that value. The expected change in light speed due to the Earth’s motion would be incredibly small.
To imagine how difficult this would be to detect, let us look at the Fizeau experiment again. A simplified schematic is illustrated below*:

In the ideal case, our entire experimental apparatus is moving parallel to the light beam on the way out, and the light is traveling at c – v. On the return trip, the apparatus is moving antiparallel to the light beam and the light is traveling at c + v. Because of these velocity differences, the round trip time the light takes will be different than that of an unmoving Fizeau experiment, by an amount:
.
Using the Fizeau experiment numbers, we find that the discrepancy would be on the order of
seconds! In order for a Fizeau-type experiment to be sensitive to that variation in time, it would have to be turning at a speed such that this time scale resulted in one ‘tooth’ rotation, or
rotations/s!
To appreciate how hopeless this is, it is to be noted that a commercial jet engine turbine has a rotation speed on the order of 36,500 rpm, or 608 rotations/second.
Is there anything that can oscillate or rotate that fast? In fact, as we have noted in an optics basics post, visible light oscillates with an angular frequency on the order of cycles/second. A light beam can therefore serve as its own ‘timer’ in light velocity measurements!
This was, in essence, the strategy employed by Albert Michelson and Edward Morley in 1887 in what is now known as the Michelson-Morley experiment. The experimental setup, of a device known as a Michelson interferometer, is illustrated below:

A light beam is incident from the left. It hits a half-silvered mirror which is inclined at a 45 degree angle, which splits the beam into two parts: one which is reflected, one of which is transmitted. Each fraction of the beam travels to a mirror and back, and at the half-silvered mirror they are recombined and their sum is projected onto a screen. Because the light has traveled a different distance in each arm of the interferometer, we get an interference pattern on the screen.
It’s worth noting that a real Michelson interferometer is a bit more complicated than the cartoon picture above, and includes a compensator plate and a lens:

These components are only present so that the behavior of the interferometer closely corresponds with the ‘ideal’, so we can neglect them in the discussion which follows.
How does this interferometer allow us, in principle, to detect the motion of the Earth in aether? (IMPORTANT: Remember, there is no aether! We’re only speaking from the point of view of what scientists of the late 1800’s believed.) We can make an analogy between light traveling in the two arms of the interferometer and boats traveling on different paths on a river*:

Each boat has a top speed of c, and the river flows to the right with velocity v. Boat 1 travels a distance d from point A to point B, and then returns, while boat 2 travels a distance d from point A to point C, and then returns. How long does it take each boat to return to the starting point? We can use a little geometry and velocity addition to determine this. Boat 2 will have a total velocity of c + v on the way out to point C, and will have a total velocity c – v on the way back. Boat 1’s velocity is slightly more difficult to calculate; in order to travel straight across the river, it must have a velocity v against the river flow, and a total velocity of c. Using some simple geometry,

boat 1 must have a speed moving from A to B, and an identical speed for the return trip. We therefore find that the transit times for the boats are as follows:
,
.
With the help of a little calculus approximation, we find that boat 1 will beat boat 2 by a time:
.
This is exactly the time discrepancy that we expected with the Fizeau experiment, as well; the difference is that with the Michelson interferometer, we are using light interference to detect this difference.
For light of angular frequency ω, the light from arm 1 and arm 2 of the interferometer will be out of phase by an amount:
.
Michelson and Morley made this shift more noticeable by rotating their entire apparatus 90 degrees; this reverses the roles of arm 1 and arm 2 of the interferometer, and results in a total phase change of
.
What did Michelson and Morley actually see? They used light from a sodium lamp (wavelength nm, angular frequency
cycles/second), a path length d = 11 meters, and with the Earth’s speed known to be
km/s, we find that we expect to see a phase change of
cycles/second.
In an actual Michelson interferometer, one actually sees a pattern of bright and dark circles of light, as shown below:

A bright spot corresponds to a phase difference of 2π, while a dark spot corresponds to a phase difference of π. When the interferometer is turned, these bright and dark circles spread outward, corresponding to a change in phase. We define the ‘fringe shift’ ΔN as the amount one dark circle moves towards the position of the next outward circle, and this shift is simply given by .
From our numbers above, Michelson and Morley expected that the motion of the Earth would result in a fringe shift of . What they in fact found, was
, which was essentially within the experimental uncertainty of their device.
In other words, Michelson and Morley could not detect any motion of the Earth with respect to the aether! This was an astonishing result. If the aether in fact existed, one could not detect one’s own motion through it, which inevitably made scientists doubt the existence of this hypothetical material.
A number of scientists tried to explain these results using arguments which can now be seen to be ad hoc: Lorentz suggested that objects moving through the aether are shrunk along their direction of motion in such a way as to equalize the time delay of light between the two arms. Others suggested that the Earth was ‘dragging’ the aether along with it, meaning that, at least locally, the aether was stationary with respect to the Earth. These suggestions, however, have an air of desperation about them, in that they raise more questions than they answer (why do objects shrink? how does the Earth drag the aether?).
It was Albert Einstein who, in 1905, proposed a new theory of relativity of such beauty and simplicity that it made the aether unnecessary. In the next relativity post, we move away from our long discussion of the historical origins of relativity and into Einstein’s amazing theory itself.


http://arXiv.org/abs/0706.2031
Michelson-Morley in 1887 nulled to 10^(-8) relative. Here it nulls to 10^(-16) relative. Optical in Berlin, Germany at 52°31’N 13°20’E and microwave in Perth, Australia at 31°53’S 115°53E. An aether background could never be at rest relative to both of them. No dichroism vs. frequency, either.
Check this for an actual application of light pulses:
http://www.3dvsystems.com/
Uncle Al: Thanks for the link; very nice! In my desire to cover only those experiments which led up to Einstein, I neglected to mention that the M-M experiment has been repeated numerous times with increasing resolution. I mentioned the most controversial of these, the work of Dayton Miller, in a much earlier post.
One loophole remains. General Relativity and string theory assume isotropic vacuum and the Equivalence Principle (all local centers of mass vacuum free fall identically regardless of composition, hydrogen atoms to neutron stars). Teleparallel gravitation wholly contains GR without isotropic vacuum or the EP. GR might detectably fail in the massed sector (but not in massless electromagetism).
Do chemically identical left and right shoes fall identically? Teleparallel theory says “no.” Spacetime torsion (replacing curvature) transforms like the Lorentz force in EM – it is chiral, a left foot. Left and right shoes fit differently. They have divergent minimum action vacuum free fall trajectories.
An Eötvös balance tests the EP to 3×10^(-14) difference/average,
http://www.npl.washington.edu/eotwash/experiments/equivalencePrinciple/epWhat.html
Click to access schlamminger08.pdf
Composition, Phys. Rev. Lett. 100 041101 (2008)
Click to access prl97-021603.pdf
Polarized angular momenta, Phys. Rev. Lett. 97 021603 (2006)
Single crystal test masses of space group P3(1)21 alpha-quartz (right-handed crew axes) opposed by space group P3(2)21 alpha-quartz (left-handed crew axes) could deeply change physics. Somebody should look.
I am curious; so can we say, 189,000 miles/sec is flawed?
Jeffrey: You’ll have to clarify what you’re asking; I’m not quite sure what you’re getting at. If you’re asking whether the results of a previous experiment which gave 189 kmiles/s is “wrong”, given what we know now, I would say not exactly: in physics all experimental results have a degree of uncertainty. 189 kmiles/s is accurate to two significant figures, i.e. the ‘180’ part of the estimate is correct.
Gravity (not gravitational waves) is the fastest thing in the universe.
Light speed is dependant on source & target (e.g., dopler) as shown in 1676, before the internet and Einstein.
Very good discussion. Thanks!
thanks for this great article!
We are forced to beleive the speed of light is constant and is independent of the relative motion between source and observer. But we could not understand it. I have framed one experiment which will show the speed of light is not constant with repsect to different observers. To built the setup a sophisticated instrumentation centre (and experts) is required.
@G H Jadhav
Pingback: Ep. 228: Giovanni Cassini | Astronomy Cast
Time is an imaginary concept
Time does not exist. It is an imaginary concept involving a method for measurement of quantities. Time does not spend. But events take place one after the other or with respect to another sequence. We count number of events with respect to spins of the standard object, “earth”.
If everything that exist in the universe maintain a state of cessation there will be no time involved. To introduce the factor “time” we should have at least one moving object.
Let there be an observer stay at an absolute location, in space. So he will see the entire universe is in motion. Earth is spinning around itself, while our galaxy is moving in another path. Each spin of earth around itself is labeled as a day on earth.
Therefore, we can create a link for an object moving in the universe, in terms of distance traveled by it, in relation to spins of earth. Earth’s spins are dimensionless, yet having a direction only, without acceleration. This link is called time.
Time 0 (zero) was assumed as 2000 spins backwards on date 2000 January 01, 0.00 Hr. at Greenwich, according to convention. Also there are other conventions for measurements of time and fixing the occurrence of events such as Lunar calendar, Persian calendar, Hindus calendar, Hebrew calendar etc.
Therefore, we have given a meaning for the sequence of events taken place, and to compare the duration and intervals using the “Time”concept, to compare the event in sequences. Time represents the idea of motion, with reference to spins of earth.
Thus time does not exist and if we imagine a factor called time we have given it a beginning yet end has not confirmed by ourselves.
Hey, Greetings. Thanks for the history lesson. I enjoyed it. — Curt 10/17/2012
Glad to hear it! 🙂
Pingback: Speed of Light | Chopping Light Beams | JAR Blog...
Could someone be so kind as to confirm the algebra is correct in the formula just after the sentence “Because of these velocity differences, the round trip time the light takes will be different than that of an unmoving Fizeau experiment, by an amount:” in the article above please? When I try to work it out I end up with negative 2 d v to the power 2 in the numerator rather than positive d v to the power 2. I appreciate there is a relatively tiny negative c v to the power 2 term that has been ‘thrown away’ from the denominator and I don’t think that is anything to do with the discrepancy I have.
Speed of Light : Reexamination
Plane waves of light (wavelength is constant) are coming from just above. An observer is moving horizontally at different speed. Speed relative to the waves does not vary. But speed relative to photons or light ray will vary (both will be real existence). With the formula : light speed = f λ, speed of waves can be shown. However, speed of photon and light ray will not be shown. Because of large speed of light, this problem is not noticeable.
In outer space, plane waves of a star light are coming. An observer is at a standstill. Speed of light waves and photons (light ray) relative to the observer will not be the same (in general). By the way, speed of light waves and of photons (light ray) relative to the aether frame will be the same (as a physical constant : not c, maybe).
Sorry, I cannot receive E-mail. I do not have PC.
http://www.geocities.co.jp/Technopolis/2561/eng.html
Constancy of speed of light
They say, it stands up on an observer in every inertial frame. Yes, when the light source shines in that frame, it is true.
Some man mistook this fact natural for a great discovery. And it is believed widely.
Really nice article, thank you. As a point of interest, does anyone know what Fizeau used as his ‘bright’ light source?